M@h*(pOet)?ica – (A Lesson in Poetic Logic–First Draft of Essay for Scientific American, if I haven’t been fired.)
This entry will be a short one in defense of the poetic logic of mathexpressive poems. By poetic logic I mean a kind of connotative, or indirect, logic as opposed (in some cases, rather extremefully) to the denotative logic of strictly scientific prose and all pure mathematics. Loose, but making intuitive sense. To show as exactly as I can what I mean, I am going to use my latest work, “Four-sided Investigation of the Core of Faereality” as an example. It consists of four long divisions of “the core of faereality.”
Before getting into my discussion of this, I think the following labeled long division example may make things go smoother (if only for me, as I still have trouble with the terminology involved!)
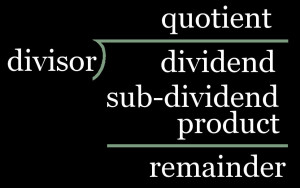
According to the above, a long division the multiplication of a long division poem’s divisor by its quotient yields what I call its “sub-dividend product” (because I’ve never been able to find its official name, if it has one). I consider this the most important operation the poem carries out because of the metaphor it makes the sub-dividend product for the multiplication of divisor and quotient, and the partial metaphor it makes it for the dividend. It is, in fact, the main point of a long division poem.
Hence, in the first long division poem in my quartet, “science,” the poem’s divisor, is multiplied by “poetry,” its quotient, to yield the sub-dividend product depicted below:

Does this make sense? It certainly does not make rigorously scientific sense, which is why mathematicians have trouble with such poetry. As a poet, though, my only concern, as previously stated, is poetic logic. I claim that science taken poetry times equals: (1) fragments of various mathematical statements because—and here you must bear with me—because poetry is in effect less that one, science more; (2) a peculiar irregular shape, because poetry is a deconstructive element; (3) a pleasurable flow of colors because of the sensually pleasing matter poetry, as a form of art, provides; and (4) the extraction from the piece’s mathematical statements a poetic text, “From is for every bound alled,” in the process uncovering a wonderful detail in a formidable set of generalizations (the definitions of elementary calculus the background text is from).
The poetic text is the most important feature of the sub-dividend product; note well, though, that it is entirely provided by the divisor; the quotient merely works out one of the many ways it can achieve poetic significance. Because of its dominance, the poetic text must be more than the weird apparently unintelligible splash of words the one here is, for the poem to give pleasure to those not aesthetically satisfied by meaninglessness. Ergo, I will try to make sense of it.
To begin with, the reader must tolerate syntactical misbehavior; to do this, he must assume that a linguistic integrity on the part of the text’s author that assures him that the author is breaking with normal syntax for some higher, poetic purpose. As that author, I claim he is! And that the reader must drop out of linguistic standardness into exploratory maples semi-dreaming. Hopefully, he will bump into the possibility that “From” not a preposition but a noun meaning “from-ness”; from there the ascent (or descent) its being for “every bound” “alled.” Paraphrase: No matter where one is coming from the result is alled, or everything possible.
At this point I need to tell you that the poem’s dividend is “the core of faereality.” Therefore, “From is for every bound alled,” according to the rules of long division, comes close to equaling that. The “bound” or territory within the core of faereality, which I use to suggest the world of the imagination, and/or the subconscious and/or where Alice went, et cetera, is what is alled.
Add the remainder, a fragment of the word, “poetry,” to the sub-dividend product and you get the core of faereality exactly. In other words, the full result of sciences taken poetry times needs a fragment of poetry to exactly equal the core of faereality. (And we have a second metaphor—one that indicates two components of the dividend, so a fuller metaphor than the ones in most ordinary poems.
There is more to the poetic text extracted than I indicated, by the way. “From” also works as a preposition, with “is” becoming a noun, and “a bound is also a leap. I hope readers will find more routes to the core of faereality (and from faereality to realms like childhood, science fantasy, dreaming, mythology, poetry (as opposed to poems), and even physics, religion and philosophy, since all of them are in some final way forms of faereality, however much else they also are.
* * *
In the quartet’s three later long divisions mathematics, poetry, and music are multiplied by poetry to yield different approximations of the core of faereality. Here’s the result of the last of poetry times music:

The only comment I’ll make about this is that I carefully removed all indications of the mathematical under-text from this (and the one resulting from poetry times poetry), since neither music nor poetry are explicitly mathematical, although both are a little mathematical, music (which has fractions!) more so than poetry. But lo, the undertext still refers back to the definitions required for calculus—the same way everything at bottom is mathematical.
.















![100_0085[1]](/wp-content/uploads/2013/11/100_00851-1024x681.jpg)
![100_0087[1]](/wp-content/uploads/2013/11/100_00871-1024x681.jpg)











i love these, bob!
Hey, thanks for the good words, Maria. As soon as I saw them, I thought of your embroidered poems and how similar my scribbled poems have in common with them–a kind of looseness, different-colored threads, domesticity (?), sensitivity (I hope!). Main thing is how much fun they are!
–Bob