Archive for the ‘Mathematical Poetry’ Category
Entry 1425 — 2 Long Division Problems
Wednesday, April 16th, 2014
I was going to send a list to an art show in Minneapolis containing mathepoetic long division problems like the two below. I couldn’t come up with enough good ones, and my list wasn’t appropriate for the show, so I sent a list of quotidian shopping list on top of notes for the list of long division problems. Here are two of said problems:

I was intrigued by the way part of the lines of the letters I used as guide for my thicker-lined spelling of “coal mine” showed through. I’d been deleting them but decided to leave them in, then wrote the crude version of “woman” on top of the violet version. It makes some kind of emotional sense to me, but I haven’t figured out what.
The problems are just multiplication problems really: what times distant cries of seagulls equals childhood (fading) and what times a coal mine will equal woman, but making them long divisions suggests the answer will have a remainder. Anyway, the idea is to get an engagent using his imagination. Simpler ones would be good exercises for children. For boy, what’s 100 zombies into interesting Sunday afternoon at the beach, maybe.
.
Entry 1342 — My Latest Poem
Thursday, January 16th, 2014
I’m going to spend the rest of the day reading a Len Deighton novel, the second in a trilogy that I’ve been reading with enjoyment this past week. I just don’t feel like any megalospiel, or even hohenspiel of any kind. Ergo, it’s pure recreospiel for me (Is that what I named it?) Nonetheless, I must post a daily blog entry, so I grabbed my latest poem for today’s:
I’m going to postpone my comments on this till tomorrow as I feel pretty sure I’ll again need something for that day’s entry, and am confident I can then at least tell you the name of the poem the above is a detail of–the remainder, actually.
.
Entry 1308 — Mine Review Continueth
Monday, December 23rd, 2013
I was going to celebrate the Winter Solstice with Zero Production, but then I found out yesterday, not today, was when it occurred, so I had to finish the review I was working on. I wrote over 1300 word, pretty good ones, I think. Below is one of the poems I dealt with, with my comments on it following it:
Something of what seems to me at the frontier of math-related poetry that I hope will be further explored in the future is Sarah Glaz’s fascinatingly strange “13 January 2009.” It consists of two texts side by side, one, “13,” with nothing in it but numbers (and equal signs), the other, “January 2009) devoted entirely to words about the dying of a man named Anuk whom I take to be an ancient Egyptian (in spite of the poem’s title!) I feel ready to go on for another thousand words at least about this poem, but will limit myself here to telling you that, according to its author, its “structure follows The Fundamental Theorem of Arithmetic, which states that every positive integer greater than one may be expressed in a unique way as a product of powers of distinct prime numbers”—which (inexorable) process, I would add, is shown in “13.” I hope to say more in an essay on mathematical poetry I have in the works for this periodical. Conclusion: when I began thinking about this review, I had visions of making an insightful taxonomical study of its poems, but their “multi-dimensional links to mathematics and . . . wide range of styles” as Glaz has it in her introduction, and wide range of techniques, I’d add, made that too difficult a task. So all I have to say now is that I hope anyone still reading this has enjoyed my chatter as much as I’ve enjoyed indulging in it.
.
Entry 1298 — “The Long Division of Creativity”
Friday, December 13th, 2013
Whee, as the year draws to a close, I finally made another long division poem:
I’ve already sketched a variation on it in which creativity is divided by summer. I hope to make a 4-season set of it.
.
Enter 1266 — A Boast
Monday, November 11th, 2013
Today, mainly once again to save work, just a short entry, this, which is from the Schirmers 2000 edition of Richard Kostelanetz’s Dictionary of the Avant Gardes, page 397:
 My boast about this is that, so far as I know, it is the world’s first definition of mathematical poetry to appear in a reputable reference. I believe it is still the only such definition to do that, certainly in the United States.
My boast about this is that, so far as I know, it is the world’s first definition of mathematical poetry to appear in a reputable reference. I believe it is still the only such definition to do that, certainly in the United States.
.
Entry 1259 — The Dead Career Goes On
Monday, November 4th, 2013
My career, by BigCity standards, may have hit rock bottom, but it CONTINUES: shown here is a wall of our county administration building with a few of the pieces in my latest local Arts & Humanities exhibition, which I hung this morning:
What the heck, here’s another wall:
.
Entry 1253 — Guest-Blog Non-Entry
Tuesday, October 29th, 2013
M@h*(pOet)?ica – (A Lesson in Poetic Logic–First Draft of Essay for Scientific American, if I haven’t been fired.)
This entry will be a short one in defense of the poetic logic of mathexpressive poems. By poetic logic I mean a kind of connotative, or indirect, logic as opposed (in some cases, rather extremefully) to the denotative logic of strictly scientific prose and all pure mathematics. Loose, but making intuitive sense. To show as exactly as I can what I mean, I am going to use my latest work, “Four-sided Investigation of the Core of Faereality” as an example. It consists of four long divisions of “the core of faereality.”
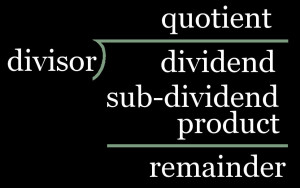
Before getting into my discussion of this, I think the following labeled long division example may make things go smoother (if only for me, as I still have trouble with the terminology involved!)
According to the above, a long division the multiplication of a long division poem’s divisor by its quotient yields what I call its “sub-dividend product” (because I’ve never been able to find its official name, if it has one). I consider this the most important operation the poem carries out because of the metaphor it makes the sub-dividend product for the multiplication of divisor and quotient, and the partial metaphor it makes it for the dividend. It is, in fact, the main point of a long division poem.
Hence, in the first long division poem in my quartet, “science,” the poem’s divisor, is multiplied by “poetry,” its quotient, to yield the sub-dividend product depicted below:
Does this make sense? It certainly does not make rigorously scientific sense, which is why mathematicians have trouble with such poetry. As a poet, though, my only concern, as previously stated, is poetic logic. I claim that science taken poetry times equals: (1) fragments of various mathematical statements because—and here you must bear with me—because poetry is in effect less that one, science more; (2) a peculiar irregular shape, because poetry is a deconstructive element; (3) a pleasurable flow of colors because of the sensually pleasing matter poetry, as a form of art, provides; and (4) the extraction from the piece’s mathematical statements a poetic text, “From is for every bound alled,” in the process uncovering a wonderful detail in a formidable set of generalizations (the definitions of elementary calculus the background text is from).
The poetic text is the most important feature of the sub-dividend product; note well, though, that it is entirely provided by the divisor; the quotient merely works out one of the many ways it can achieve poetic significance. Because of its dominance, the poetic text must be more than the weird apparently unintelligible splash of words the one here is, for the poem to give pleasure to those not aesthetically satisfied by meaninglessness. Ergo, I will try to make sense of it.
To begin with, the reader must tolerate syntactical misbehavior; to do this, he must assume that a linguistic integrity on the part of the text’s author that assures him that the author is breaking with normal syntax for some higher, poetic purpose. As that author, I claim he is! And that the reader must drop out of linguistic standardness into exploratory maples semi-dreaming. Hopefully, he will bump into the possibility that “From” not a preposition but a noun meaning “from-ness”; from there the ascent (or descent) its being for “every bound” “alled.” Paraphrase: No matter where one is coming from the result is alled, or everything possible.
At this point I need to tell you that the poem’s dividend is “the core of faereality.” Therefore, “From is for every bound alled,” according to the rules of long division, comes close to equaling that. The “bound” or territory within the core of faereality, which I use to suggest the world of the imagination, and/or the subconscious and/or where Alice went, et cetera, is what is alled.
Add the remainder, a fragment of the word, “poetry,” to the sub-dividend product and you get the core of faereality exactly. In other words, the full result of sciences taken poetry times needs a fragment of poetry to exactly equal the core of faereality. (And we have a second metaphor—one that indicates two components of the dividend, so a fuller metaphor than the ones in most ordinary poems.
There is more to the poetic text extracted than I indicated, by the way. “From” also works as a preposition, with “is” becoming a noun, and “a bound is also a leap. I hope readers will find more routes to the core of faereality (and from faereality to realms like childhood, science fantasy, dreaming, mythology, poetry (as opposed to poems), and even physics, religion and philosophy, since all of them are in some final way forms of faereality, however much else they also are.
* * *
In the quartet’s three later long divisions mathematics, poetry, and music are multiplied by poetry to yield different approximations of the core of faereality. Here’s the result of the last of poetry times music:
The only comment I’ll make about this is that I carefully removed all indications of the mathematical under-text from this (and the one resulting from poetry times poetry), since neither music nor poetry are explicitly mathematical, although both are a little mathematical, music (which has fractions!) more so than poetry. But lo, the undertext still refers back to the definitions required for calculus—the same way everything at bottom is mathematical.
.
Entry 1228 — Protozoa, Part 2
Sunday, September 29th, 2013
Here is the corrected version of my piece in Shadows of the Future (it’s now in the latter, too, thanks to Jeff Side):
I’m still too blah to say why the change was (vitally) necessary, but I told Jeff, when asking him if he could switch versions, that, for me, it would be like changing “5 x 10 = 2″ to “5 x 2 = 10″–which should give you a huge clue as to my thinking.
And now, from Wikipedia, to make this the first blog in history to contain an entry on mathematical poetry and protozoa, is some information about the flagella of protozoa:
Types
Three types of flagella have so far been distinguished; bacterial, archaeal and eukaryotic.
The main differences among these three types are summarized below:
- Bacterial flagella are helical filaments, each with a rotary motor at its base which can turn clockwise or counterclockwise. They provide two of several kinds of bacterial motility.
- Archaeal flagella (Archaella) are superficially similar to bacterial flagella, but are different in many details and considered non-homologous.
- Eukaryotic flagella – those of animal, plant, and protist cells – are complex cellular projections that lash back and forth. Eukaryotic flagella are classed along with eukaryotic motile cilia as undulipodia to emphasize their distinctive wavy appendage role in cellular function or motility. Primary cilia are immotile, and are not undulipodia; they have a structurally different 9+0 axoneme rather than the 9+2 axoneme found in both flagella and motile cilia undulipodia.
Bacterial
Physical model of a bacterial flagellum
Structure and composition. The bacterial flagellum is made up of the protein flagellin. Its shape is a 20 nanometer-thick hollow tube. It is helical and has a sharp bend just outside the outer membrane; this “hook” allows the axis of the helix to point directly away from the cell. A shaft runs between the hook and the basal body, passing through protein rings in the cell’s membrane that act as bearings. Gram-positive organisms have 2 of these basal body rings, one in the peptidoglycan layer and one in the plasma membrane. Gram-negative organisms have 4 such rings: the L ring associates with the lipopolysaccharides, the P ring associates with peptidoglycan layer, the M ring is embedded in the plasma membrane, and the S ring is directly attached to the plasma membrane. The filament ends with a capping protein.
The flagellar filament is the long helical screw that propels the bacterium when rotated by the motor, through the hook. In most bacteria that have been studied, including the Gram negative Escherichia coli, Salmonella typhimurium, Caulobacter crescentus, and Vibrio alginolyticus, the filament is made up of eleven protofilaments approximately parallel to the filament axis. Each protofilament is a series of tandem protein chains. However in Campylobacter jejuni, there are seven protofilaments.
The basal body has several traits in common with some types of secretory pores, such as the hollow rod-like “plug” in their centers extending out through the plasma membrane. Given the structural similarities between bacterial flagella and bacterial secretory systems, it is thought that bacterial flagella may have evolved from the type three secretion system; however, it is not known for certain whether these pores are derived from the bacterial flagella or the bacterial secretory system.
Motor. The bacterial flagellum is driven by a rotary engine (the Mot complex) made up of protein, located at the flagellum’s anchor point on the inner cell membrane. The engine is powered by proton motive force, i.e., by the flow of protons (hydrogen ions) across the bacterial cell membrane due to a concentration gradient set up by the cell’s metabolism (in Vibrio species there are two kinds of flagella, lateral and polar, and some are driven by a sodium ion pump rather than a proton pump). The rotor transports protons across the membrane, and is turned in the process. The rotor alone can operate at 6,000 to 17,000 rpm, but with the flagellar filament attached usually only reaches 200 to 1000 rpm. The direction of rotation can be switched almost instantaneously, caused by a slight change in the position of a protein, FliG, in the rotor.
The cylindrical shape of flagella is suited to locomotion of microscopic organisms; these organisms operate at a low Reynolds number, where the viscosity of the surrounding water is much more important than its mass or inertia.
The rotational speed of flagella varies in response to the intensity of the proton motive force, thereby permitting certain forms of speed control, and also permitting some types of bacteria to attain remarkable speeds in proportion to their size; some achieve roughly 60 cell lengths / second. Although at such a speed it would take a bacterium about 245 days to cover a kilometre, and although that may seem slow, the perspective changes when the concept of scale is introduced. In comparison to macroscopic life forms it is very fast indeed when expressed in terms of number of body lengths per second. A cheetah for example, only achieves about 25 body lengths / sec.
Through use of their flagella, E. coli are able to move rapidly towards attractants and away from repellents. They do this by means of a biased random walk, with ‘runs’ and ‘tumbles’ brought about by rotating the flagellum counterclockwise and clockwise respectively.
Assembly. During flagellar assembly, components of the flagellum pass through the hollow cores of the basal body and the nascent filament. During assembly, protein components are added at the flagellar tip rather than at the base. In vitro, flagellar filaments assemble spontaneously in a solution containing purified flagellin as the sole protein.
Evolution. The evolution of bacterial flagella has been used as an argument against evolution by creationists. They argue that complex structures like flagella cannot evolve from simple structures. In other words, flagella are “irreducibly complex” and need all of their protein components to function. However, it has been shown by numerous studies that a large number of proteins can be deleted without (complete) loss of function. Moreover, it is generally accepted now that bacterial flagella have evolved from much simpler secretion systems, such as the Type III secretion system.
* * *
Danged inneresting, I think!
.
Entry 1227 — A New Anthology
Saturday, September 28th, 2013
Yesterday Jeff Side announced the e.publication of Shadows of the Future, an anthology of otherstream poetry (or, in some cases–in my possibly excessively picky opinion–almost otherstream poetry) edited by Marc Vincenz, and published by Argotist Ebooks. So I’m going to use this entry for some words about it instead of going on to the second part of my investigation of protozoa. That I will do tomorrow, assuming I choose to continue my investigation (and I hope I do–nothing more valuable for the ol’ brain than a plunge into something you don’t know hardly nothin’ about).
Interesting. When looking for what categories to assign this entry to, I found I had none for “Poetry.” I do now. So I can bring up that subject to tell you the anthology has 120 works, almost all of them poems by my definition, on 166 pages . . .
and here a digression to complain about my stupid computer (or, yeah, my stupid inability to know how use it): I would like to be able to click from here to the anthology the way I can click from here to a file on my word processer or anywhere else but totally out of it. There must be a way to save it as a regular file I can access on my word processer; if so, I’m ignorant of it. So I have a second copy of this entry on the slot (can’t remember its name) with everything I can click to on it. To get to the anthology, I go to that entry, and click the link in it to the anthology. Very annoying.
Back to the anthology now. Marc has a nice one-page forward in it. Following it is a page-and-a-half introduction to it by me which is just my standard boilerplate about the refusal of the Establishment to so much as acknowledge the existence of the Otherstream. Basically it’s a polemic intended to annoy estabniks enough to make them reply to it. It has little chance of doing that but what else can I do? I think it presents a good definition of the establishment, though.
My only real disappointment with the anthology is how little visual poetry is in it–but that was because, for some reason, few visual poets submitted anything to it. There were visual poems by seven people–and textual poems by five people like mIEKAL aND who often do visual poetry. In all, 37 had works in the anthology. When going through it doing my counts, I spent a few minutes with my own poems. One of them disturbed me: I decided it was wrong! Here is the wrong version:
I doubt anyone but I would see what is wrong (crucially wrong, in my view) with this, but just for the fun of it, I won’t say more about it, nor show the corrected version for a while.
I’m too worn out from being too worn out to say much more about the anthology. Before signing off, though, I want to recommend it strongly. It’s an excellent tour of what’s going on in the vast countryside beyond the borders of the mainstream. The vispo cover by David Chirot is worth the trouble of clicking to it alone! That one work will give you more to wander through thoughts and feelings about by itself than the entirety of most mainstream anthologies of contemporary poetry.
.
Entry 1205 — The Experioddicist, July 1993, P.2
Friday, September 6th, 2013




![100_0085[1]](/wp-content/uploads/2013/11/100_00851-1024x681.jpg)
![100_0087[1]](/wp-content/uploads/2013/11/100_00871-1024x681.jpg)